The Question of Vertical Exaggeration [1]
Vertical exaggeration describes the fact that three-dimensional representations of the earth’s surface often are modeled in two different scales: The horizontal scale is often smaller than the vertical scale; that is, the mountains are too high in the model as compared to the real terrain. Exaggeration helps depict mountains in small scale models, otherwise they will be too flat and the typical shaping of a landscape cannot be shown. Without exaggeration, Mont Blanc (4807 m) corresponds to an elevation of only 5 mm in a relief with a scale of 1:1,000,000.
Exaggeration is normally indicated by the ratio of vertical scale to horizontal scale. An exaggeration of 2:1 means that the mountains are twice as high as they were if their elevation had been left unchanged.
As already postulated by Albert Heim (1849–1837), models of mountainous and hilly regions in large scale should never be exaggerated. But for small scale models or flat terrain, it is inevitable to exaggerate the third dimension. Normally, terrain models with a scale smaller than 1:50,000 are exaggerated. Vertical exaggeration also depends on the relief energy of the depicted landscape. A flat countryside must be more exaggerated than mountainous regions. The smaller the scale, the more extensive the exaggeration.
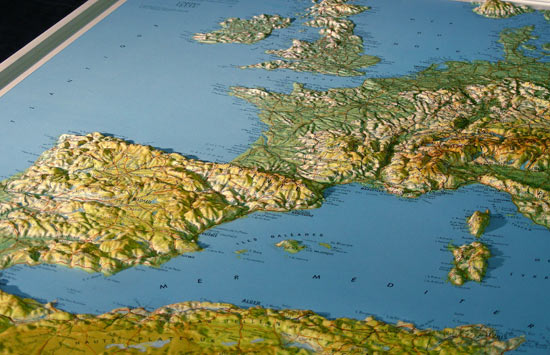
Relief of Europe 1:5,000,000, vertical exaggeration 12.5:1 to 25:1,
Map collection ETH, Zurich
The magnitude of the exaggeration is partly a matter of taste; the opinions of different authors diverge. Common exaggeration factors range from about 1.2:1 up to extremely exaggerated elevation on relief globes of perhaps 100:1. Normal values are within 2:1 to 10:1 depending on scale and landform.
Every exaggeration distorts the geometrical form. Thus, models created for geomorphological studies should show the same scale in vertical as in horizontal dimension.
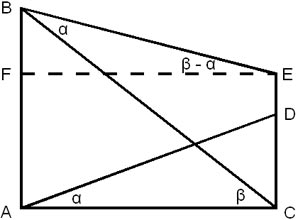
This figure shows why a slight exaggeration can be useful according to Albrecht Penck: The viewer’s eye at point B sees the distance C – E (exaggerated relief) in the same angle α as an observer in nature would see a mountain of the height of D viewed from A (E: height of the exaggerated relief, D: height of the unexaggerated relief).
Reliefs incorporating the earth’s curvature
Terrain models at small scales, covering a large part of the earth’s surface, should take the spherical curvature of the earth’s surface into account. This is the only way to avoid distortions. When terrain models are used for exact measuring purposes, it is indispensable to reproduce the earth’s curvature. Such a relief represents a segment of the globe.

Geologically painted relief of Switzerland with incorporated earth’s curvature without exaggeration 1:100,000, 259×359 cm, Charles Eugène Perron, 1893–1900,
Collection, Department of Earth Sciences, ETH Zurich
Examples of terrain models with incorporated earth’s curvature are the model of Italy in 1:1,000,000 created by Cesare Pomba in 1884 [2] and the relief of Switzerland in 1:100,000 by Charles Eugène Perron (1837–1909) [3]. During World War II, Karl Wenschow (1884–1947) refined his pantograph machine to create models accounting for the spherical curvature of the earth’s surface [4].
[1] See about this topic:
- Gygax, Fritz. Das topographische Relief in der Schweiz. 1937. S.10,11.
- Imhof, Eduard. Bildhauer der Berge. 1981. S.149.
- Mühle, Helmut. Manuelle Geländemodell-Herstellungsverfahren unter Berücksichtigung des Standes der Technik. 1963. S.41,42.
- Penck, Albrecht. Neue Reliefs der Alpen. 1904. S.98,99.
[2] Penck, Albrecht. Pomba’s Relief von Italien. 1890. S.50.
[3] Imhof, Eduard. Bildhauer der Berge. 1981. S.133.
[4] Harrison P.. The development of terrain model in the war. 1946. p.650.
